|
“Estrella” - composition analysis |

|
Chapter 2. Nested or recursive system |
|
© V.Kulikov |
|
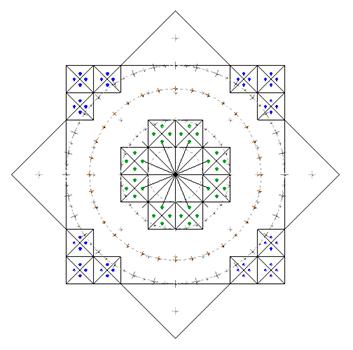
Fig. 1 With the addition of the circles, the system gets a new impact for the deformations. On the background of the circle, the weakness of the orthogonal system against the symmetry is forcing it to modify its internal structure of the elements by adding new rules in their relations.
With the preservation of the modular structure, the square will get closer to a circle: with the decreasing of the cell size, the ability to create a variety of the equidistant from the center elements - increases.
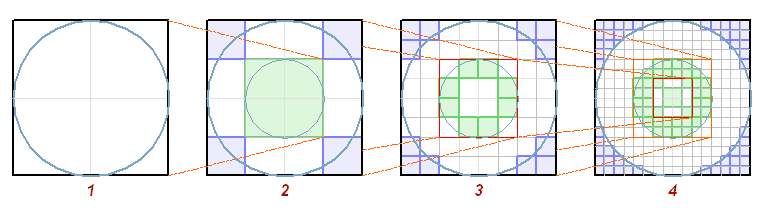
Fig. 3 Lets pay attention to the Stage 2 and 3 of the repeated process of dividing the modular cell.
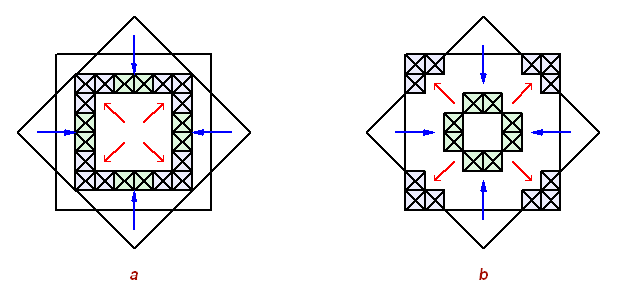
Fig. 4
Fig. 5 The fact, that the composition is consisting of the elements, which are identical to the structure of the general composition, indicates on its recursive properties. The fact, that nested compositions are representing that very general system at the previous stage of its development, indicates on the internal dynamics of a fractal process. The result of this process could be a certain goal, located "beyond the infinity". For example: The number of the cells inside the circle will seek to the circle area, and the number of adjoining outside cells will seek to the outside border of the circumference. These values are associated with the linear size of the circle radius by an irrational number Pi, and therefore the exact result is impossible in this case. The process, directed to the infinity by means of dividing the modular grid, will approximate to the true circle with each iteration. I think this is a very important layer of the drawing. If we assume that it is also has an illustrative finction ( from the inheritance of the parent process), we can make the following conclusion: The drawing is representing itself the repeating processes of specification or discretization, which are endlessly approximating to the infinitely remote solution. According to the general rules, working within the system, it means, that other elements of the system should be considered within the dynamics of the repeatable processes. The composition contains the items, which are existing in the direct relation between each other. The rays, the marking dots and the marked cells are the members of the same compositional theme. Is that a simple coincidence? Being superimposed on a single logical basis, it can not be just a coincidence. The clearly defined "sign function" of the auxiliary elements of the scheme tells us, that someone wanted to draw our attention to the reading of geoglyph's geometry. To speak of Estrella, as about just an aesthetic research in the field of "geometric ornamentology", is very, very difficult. I feel ill at ease to write about the discretization of a fractal approach, examining a group of geometric primitives of the primitive times. Who could so easily manipulate with the multi-level geometric concepts, placing ten-meter crosses in the cells, on the distances of several hundred meters? Who is that "someone", who was trying to illustrate something, so deeply taking care for it's understanding? .. Whether all these coincidences are occasional? I just try to follow what I'm am seeing by my own eyes. It's like finding a figure of the Bear among the billions of stars. But the geoglyphs provides many regularities, and some of them are brighter and grouped in a certain lines, thus making this "Bear" to became a volumetric model, walking around us. Of cause, one can regard it as a pleasant hallucination, but it seems to me, that one better pick up his legs, not letting this Bear accidently to step on his foot. |
|
Ggeoglyphs in the Nazca mountains - is it just a simple ornament or the plan |