In 1992 and 1993 I had the opportunity of undertaking three archaeological campaigns on the Cheops Pyramid in collaboration with the German Archaeological Institute in Cairo.  An important objective of the work then undertaken was to re-survey certain important basic points within the corridor chamber system.  I had noticed that several measurements that I required to produce a digital computer model were incomplete or wrong and a re-survey was therefore required.  I had for some time been considering the extent to which the measurements obtained from a structure permit conclusions as to the construction stages applied.  In doing so, I assumed that present day designs by engineers or architects also contained traceable construction stages which quite substantially led to conclusions as to the basic thinking behind the design.  In addition, the analysis of whole-number values is of decisive importance.

An example will make this clear.  A present-day architect is hardly likely to plan a house from the start which is 20.63 m long and 10.95 m wide but if we follow the rules of logic will initially have a house of 11 by 20 meters in mind.  Even if a whole-number criterion of this kind could not be put into practice on structural grounds, the first mental step is nonetheless always in whole figures.  When I find whole-number values in a structure, they must essentially be regarded as primary construction steps to which the non-whole-number, secondary construction steps are subordinate.

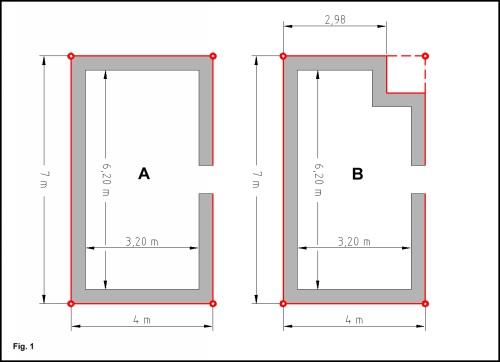
Let us take the simplified layout of a modern house as an example (Fig. 1A).

Back to the text position

We can already derive some information from it.  The architect has worked not with inches but with meters and has conceived it from the outside inwards.  No precisely defined spatial requirement or, possibly, the given length of a piece of furniture predominates, but the layout of the house itself.  It therefore forms the architect's first design step.  The points relevant to the structure lie at the four corners of the building.  The dimensions of the internal space, on the other hand, are obtained only through structural components, namely the requisite wall thickness.  The internal space is consequently a secondary design product which is already subject to structural constraints.  If we follow the simple and proven principle, the whole-number values can also be set out as grids.  Whole-number planning is evident in a great many Egyptian structures (e.g. in the Oracle Temple in Siwa).  However, 100% correspondence is not always evident. There are a number of possible causes for this:

1. inaccuracies in the means of measuring methods then used.
2. imprecise execution of the works 
3. changes in plan during construction.
4. regional departures in the unit of measurement
5. geological shifting (e.g. the Oracle Temple in Siwa).

These factors necessitate a certain tolerance in calculating, corresponding to the general structural performance of the culture concerned.However, these should be kept well beneath the limit where all dimensions become freely interpretable quantities.  In the case of the Cheops Pyramid, I allowed a maximum departure from the grid of 5cm.  This extremely small value was possible only through the precise execution of the work on this pyramid.  In addition, the grid had to be calibrated to the unit of measurement actually applied.  This means that the mean of the longest sections to be assumed as whole-number (in the case of the Cheops Pyramid, the pyramid base) is divided by the value that the builder aimed for.  Since the master builder of the Cheops Pyramid will hardly have laid out the base as 439 x 439 or 441 x 441 cubits, we must assume that the basic dimension he had in mind was 440 x 440 cubits, which brings us to a unit generally recognised for Cheops of 1 cubit = 0.5236 meters.  In the case of the Cheops Pyramid, the process comes up against further limits, imposed by the size of the structure. A grid of 440 x 280 cubits can no longer be precisely set out on paper, even in format A0, since simply the shrinking of the paper through humidity can make a whole cubit´s difference. This task can therefore only be executed on the computer, i.e. by precise mathematical visualisation.  I have produced a three-dimensional model for this on the CAD system AUTO-CAD; it contains the measurement results of Petrie, Maragiolio and Rinaldi, the Edgar Brothers and the data I ascertained for myself in 1992 and 1993.  This model is therefore the best and most exact basis at present for ascertaining points within the Cheops Pyramid relevant to its design.  The illustrations given below are based on this computer model.  However, before we can now start with an evaluation, i.e. the search for whole-number values and ratios, we must know which points were of special importance within the structure, namely at which points were heights or distances actually measured during construction?

This is a search process for structural indices which I should like to clarify here by means of selected examples.  Fig. 2 shows the northern wall of the Queen's Chamber. 

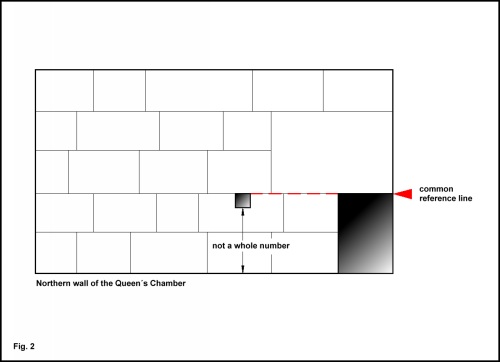
Access to this chamber and the entrance to the northern air shaft have only one reference in common, namely the same height of the ceiling.  The distance between the floor in the chamber and the floor of the shaft, on the other hand, is not a whole-number value.  We find the same in the King's Chamber.  This initial reference to the fact that measurements were taken at the ceiling or practically before the corridor was covered over, on the smooth upper surface of the side walls free of building debris, is confirmed at further places in the structure.  The ceiling height similarly constitutes a common quantity at the lower end of the Great Gallery (Fig. 3).

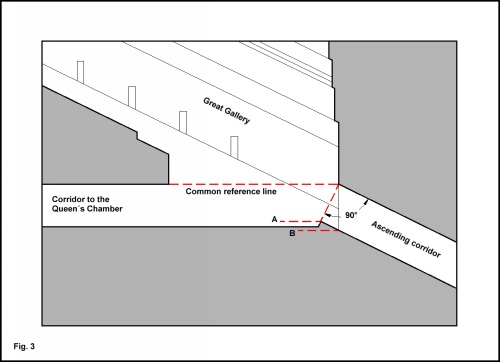
At the floor, on the other hand, neither the 90° angle A towards the landing nor the vertical B of the jointing forms a defined common intersection of the three structures.  Since the heights of the two galleries are different, there is certainly no common intersection at the angle bisection, even if one were to assume that the ancient Egyptians were in a position to determine the bisector.  We obtained the same picture at the upper end of the Great Gallery (Fig. 4).

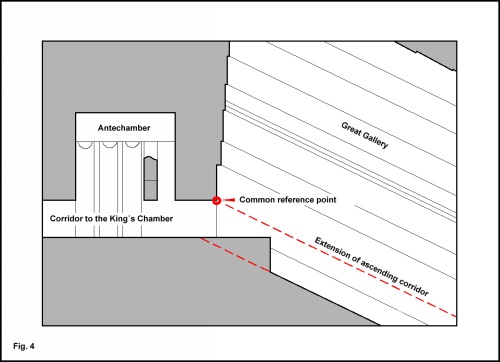
A clearly defined, common reference point for all three structures lies in the height of the corridor ceilings. On the floor, however, there is no defined conclusion, either for the Great Gallery nor for the other galleries. Here, too, the corridors, or their theoretical extension, do not have the same heights, so that here no conclusion is possible at the angle bisects, either. A further indication that the ceiling height was very probably a primary construction quantity is evident from the Cheops Pyramid's rock chambers. The ceilings are the only completed element of these chambers, a finding which occurs also in other rock chambers of the Old Kingdom. The walls and floor, on the other hand, are still in unfinished state and cannot therefore possibly have served as a reference for measurements.

These indices point to the fact that the primary construction points must generally be looked for at the ceilings and not on the floors, which hitherto have simply been regarded as a reference height for no good reason. The indices that we have compiled so far are highly meaningful, but they still do not amount to proof. The thesis is proved only when the analysis of the points ascertained in this way reveal meaningful design steps and a thought-out system. If all the structural points ascertained in the past are entered into a computer model of the Cheops Pyramid reduced to essentials, they all end up as whole numbers (shown in red, see Fig. 5).

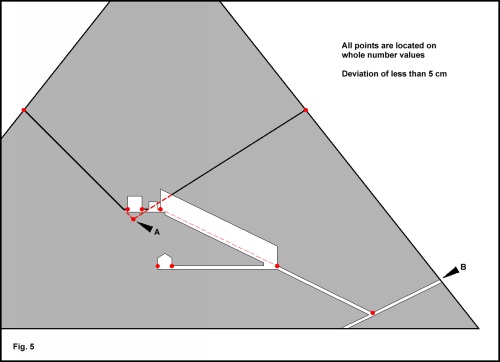
Even the lower intersection of the upper air shafts A, even though only theoretically existing, forms a whole number.  At the exit B of the ascending corridor from the pyramid, the intersection is not a whole number.  We have to take one step back to understand the reason for this. 


The example of the simplified, modern house layout   (Fig. 1B) shows that the upper left reference point is not technically executed although it was needed both for the paper design, because after all one logically starts here by drawing a rectangle, and for measurements during construction.  Could the missing point for the Cheops Pyramid therefore also lie outside the structure?  Crossing of the paths with the missing point is shown in Fig. 6

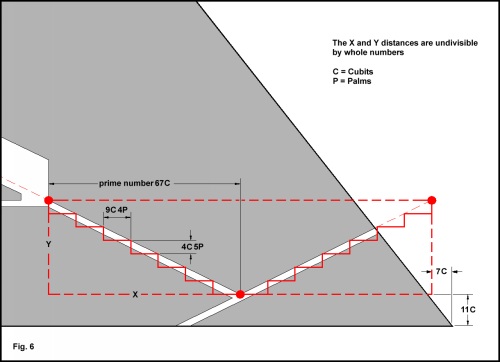
Both corridors have exactly the same angle, so that what applies to the ascending one must also apply to the descending one. The length of the X coordinate is a prime number in the ascending corridor. Because this is indivisible, the only possibility of nonetheless obtaining a division into easily measured sub-quantities is to move down into the next smaller measurement unit.

With the Egyptian cubit, this is the so-called "palm" of 4 fingers. This division, which looks like a staircase in the illustration, clearly shows that the diagonal XY possesses only two whole-number points, namely the commencing point and the terminal point. We can also see that even moving down into the next smaller measurement unit does not create an intersection point with the pyramid's flank, which in any event could never coincide with a whole-number cubit value.

A further movement back into the finger measurement unit therefore makes little sense and, as I have checked, similarly fails to produce a definable crossing point. We must therefore admit here that the Egyptians of the Old Kingdom simply did not master the intersection of three different angles and they were clearly compelled to place the imaginary structural point outside. At the base of the pyramid, the result is therefore a shift from 7 to 11 cubits, a quantity that we shall encounter again in due course. Now that we have found the last, missing point, we can start on the evaluation.

Fig. 7 shows that the same quantities occur time and again in the distances between the whole-number construction points. Distances of the same length are in each case shown in the same colour. The quantities are repeated up to three times and, with the exception of two values, are all divisible by 11. This first reference to an existing system in planning the pyramid is impressively confirmed when analysing the heights of the construction points (Fig. 8).

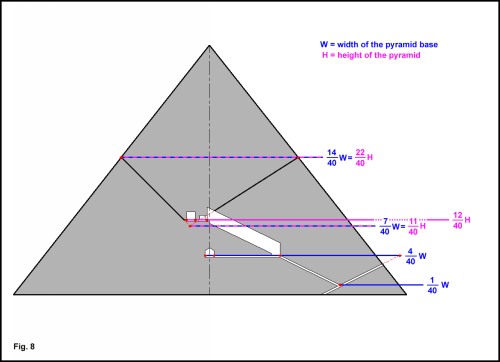
The heights of all construction points are essentially either one 40th of the basic width of the pyramid or one 40th of their height or, in two cases, even one 40th of both (these designate the construction points of the upper air shafts). This is all the more surprising when we consider that the base of 440 cubits when divided by 40 produces 11 and the height of 280 cubits when divided by 40 produces 7. Two exceptions apart, as we have already seen, all distances of the same length are divisible by 7 or 11 or both. This clear sub-division into 40ths leads us to suspect that the architect of the Cheops Pyramid worked in a scale of 1:40 when placing his plan on papyrus (?). Especially significant, however, is the fact that the construction points ascertained are clearly really relevant to the design, i.e. the steps that we have taken to discover them were the right ones. After having first created the basis for understanding the structure of the Cheops Pyramid, I should finally like to show, by means of an example, how our knowledge of the points relevant to the design can be put to use. In our measuring campaigns in 1992, I attached particular value to measuring the exit points of the upper airshafts. Although the surveys by Maragiolio and Rinaldi showed only poorly measured quantities, even with confusion between south and north, I could already clearly see that these exit points lay at the same height (Fig. 9).

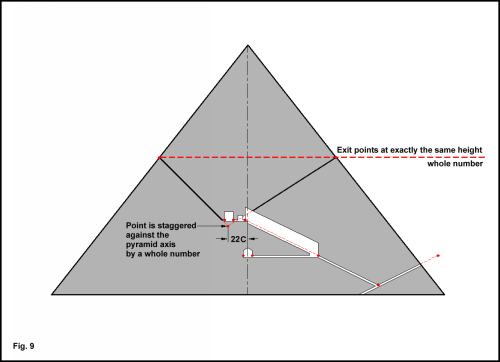
Our measurements confirm this suspicion, the departure from the whole-number cubit grid remaining within the tolerance limits set. Since the theoretical, lower intersection point of the shafts is also staggered against the axis of the pyramid by a whole number, it is clear that the Bauval theory that these shafts were aligned on certain stars does not add up. The shafts are clearly designed by whole numbers and according to clear geometrical rules. On their being aligned on certain stars, the same exit height would, moreover, be all too much of a coincidence. It is interesting that the shifting of the lower construction point of the shafts from the pyramid axis amounts to exactly 22 cubits, i.e. 2 x 11 cubits. This shift resulted in quite substantial problems during execution of the works, because the exit points clearly had to lie at equal height. For this, not only had two angles to be determined but so had the ratio of the two angles to each other and to the axis of the pyramid, in order for them to be precisely executed structurally.

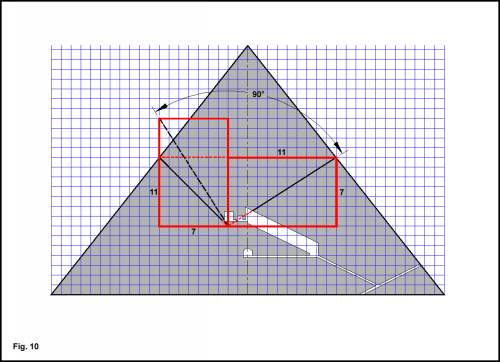
Fig. 10 shows the mathematical geometrical principle with which this problem was easily solved. A grid of 11 x 11 cubits was placed above the pyramid. The grid therefore corresponds to a scale of 1:40 referred to the pyramid base. This grid is irrelevant to the height of the pyramid. In actual fact, the Cheops grid, as I ascertained during my ongoing work, is not square but rectangular, in a ratio of 7 to 11 cubits, i.e. one 40th of the height to one 40th of the base. We are using the square grid here only to clarify the design process more effectively. The right northern shaft is clearly designed in a ratio of 11:7 grid points and the left southern one in a square ratio of 7:7 grid points. By reversing the ratio of 11:7 to 7:11, I obtained the counter-angle in the diagonal (shown by a broken line), which lies at 90° to the northern shaft. The angle, the counter-angle and the square counter-ratio can therefore be geometrically determined. When we remember that the ancient Egyptians had to rely on only imperfect drawing aids when planning this gigantic structure, i.e. when drawing they could not determine with sufficient precision in a grid whether the line drawn and the grid actually intersected or only seemed to do so through the small scale, we can see that draughtsmanship alone was insufficient to enable such precise building work. An additional means was therefore needed to prove the assumed intersecting points mathematically. We do know from the Rhind Papyrus that the ancient Egyptians calculated with fractions. I believe that this simple, mathematical knowledge can also be imputed to the Old Kingdom. Ratios of the kind arising with the shafts can of course also be expressed in fractions. In doing so, the X quantity forms the denominator and the Y quantity the numerator. We therefore say that 11/7 forms the counter-angle of 7/11 (cf. Fig. 10).

A simple arithmetical operation (Fig. 11) which today curiously can only be found in alchemical literature and over which every mathematician only shakes his head, suddenly becomes a simple method of bisecting the angle when geometrically demonstrated. We are no longer familiar with this process today, because we have, in the meantime, as we know, discovered far better methods for bisecting the angle.

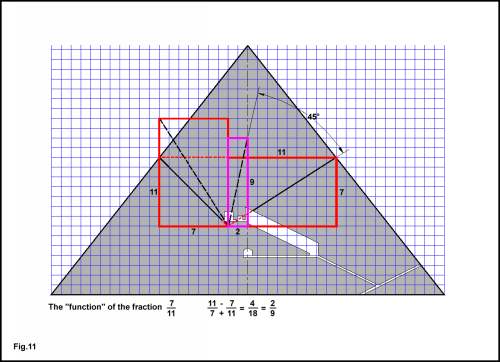
Subtracting the numerator from the ratio and counter-ratio with simultaneous addition of their denominators in principle always produces the bisector of the angle when geometrically demonstrated. The ancient Egyptians seem to have known this process, since the bisector of the angle or, to put it more precisely, the functional ratio of 2:9 grid points indisputably defines the shift of the shafts from the axis of the pyramid. 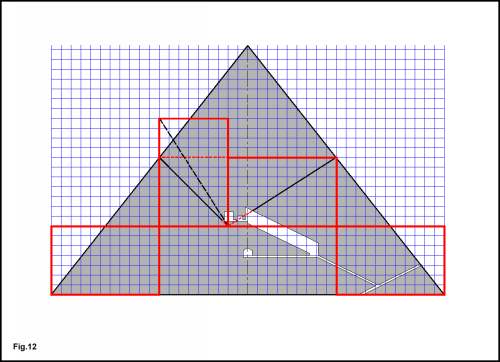As we can see from Fig. 12, the lower part of the pyramid right down to the base logically continues to sub-divide in the ratio 7:11.

I believe that the finger print of the architect of the Cheops Pyramid now becomes clearly visible. Already at this stage, he allows us to draw conclusions with far-reaching consequences. The master builder of the Cheops Pyramid was familiar neither with Pythagoras' theorem nor that of Tange, or they would otherwise have made use of this knowledge instead of messing about with primitive mathematical systems.

On the other hand, it is also clear that they did not embark on a reckless building spree but that the structure was already carefully planned before work commenced, with the consistent application of expertise that was still relatively simple for the period. We also discovered that the upper air shafts mark the commencement of interior design because their construction, contrary to all other spatial elements, remains whole-numbered right down to the smallest possible scale. As I have intimated above, simply creating the right basic prerequisites for analysis is itself a thoroughly rewarding process. The quantities relevant to the structure must first be separated from those which are of only secondary importance to the building work, since only then can the contrasting of unrelated quantities be prevented, like comparing chalk with cheese. I hope that with this paper I have made a small contribution towards lifting the structure of the Cheops Pyramid out of the numerically esoteric taboo area of science and restoring it to what it really is, namely one of the most informative structures of antiquity.